Second Order Circuits: Difference between revisions
No edit summary |
|||
| Line 10: | Line 10: | ||
= Series RLC Circuits = | = Series RLC Circuits = | ||
== | == Natural Response == | ||
[[File:Unforced RLC Circuit.png|thumb|An unforced series RLC circuit]] | [[File:Unforced RLC Circuit.png|thumb|An unforced series RLC circuit]] | ||
Consider an un-forced RLC circuit. We want to find <math>V_C</math>. | Consider an un-forced RLC circuit. We want to find <math>V_C</math>. | ||
| Line 34: | Line 34: | ||
= Parallel RLC Circuits = | = Parallel RLC Circuits = | ||
== | == Natural Response == | ||
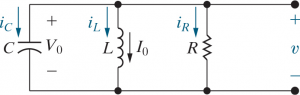
[[File:Parallel Unforced RLC Circuit.png|thumb|A parallel unforced RLC circuit]] | [[File:Parallel Unforced RLC Circuit.png|thumb|A parallel unforced RLC circuit]] | ||
[[Category:Electrical Engineering]] | [[Category:Electrical Engineering]] | ||
Revision as of 06:56, 8 March 2024
Second order circuits are circuits that have two energy storage elements, resulting in second-order differential equations.
One application of second order circuits is in timing computers. As we will see, an RLC circuit can generate a sinusoidal wave.
There are primarily two types of second order circuits:
- Parallel RLC circuits
- Series RLC circuits
Series RLC Circuits
Natural Response
Consider an un-forced RLC circuit. We want to find Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle V_C} .
First, we can use KVL and KCL
Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle V_R + V_L + V_C = 0}
Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle iR + L \frac{di}{dt} + V_C = 0}
Next, we can use Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle i = C \frac{dV_C}{dt}} and substitution to get
Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle RC \frac{dV_C}{dt} + L \frac{d}{dt} \frac{C V_C} {dt} V_C = 0}
Changing the order and moving the constants,
Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle LC \frac{d^2 V}{dt^2} + RC \frac{dV_C}{dt} + V_C = 0}
Moving constants away from the first term to get a second-order differential equation,
Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \frac{d^2V_C}{dt^2} + \frac{R}{L} \frac{dV_C}{dt} + \frac{1}{LC} V_C = 0}
Parallel RLC Circuits
Natural Response
